In Part 1 we discussed the process wheel and the first two elements of that wheel, namely candidate selection and problem clarification. In Part 2 we will focus on generating a connection between the problem types as outlined in the conformance problem matrix and various methods of trying to solve these problems.
In this section we will continue to utilize the conformance problem matrix outlined in Part 1 and incorporate various overlays on this matrix to describe how elements of the conformance solution continuum and the subsection of the continuum, i.e., existing wellbore interventions, can be used to show how these solutions can be effectively applied.
Defining the Problem Matrix
Prior effort to classify conformance engineering problems have used a linear view of grading problems from easiest to most difficult to control. Probably the most quoted document in this effort is by Seright et al., SPE 84966. Although I don’t disagree with their ranking process, it really doesn’t help us to focus on the most appropriate solution.
A different way of classifying conformance problems was developed, briefly described, and presented in Part 1. The conformance problem matrix (Fig. 1) allows us to classify conformance problems based on two primary problem characteristics: the dominant problem flow path (i.e., a VSC [void-space conduit] or permeable rock) and the location of prominent flow control (i.e., near the wellbore or deeper in the reservoir). Although some problems can contain multiple characteristics, the key is to define the most dominant characteristic so it can be solved first.
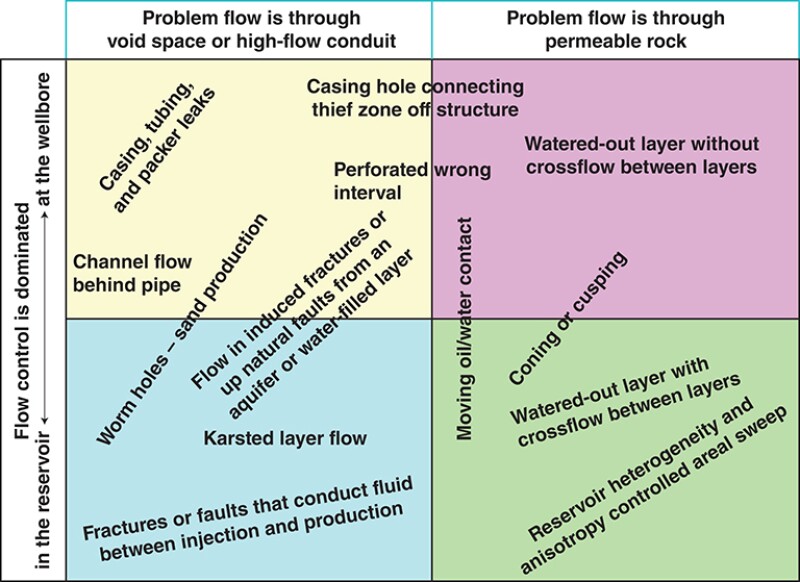
Not every conformance problem is presented in this chart, but if you consider these two major elements of the problem flow, we should be able to place any type of conformance problem on this matrix. Please study and consider this matrix carefully since this will be a key element of Part 2.
In Part 2, to be published in the June JPT, we will provide a more in-depth discussion on the problems and the problem matrix. We will also discuss wellbore intervention solutions and how to overlay these solution types on top of the conformance problem matrix. Additionally, we will introduce the conformance solution continuum and show its relationship to the problem matrix.
Populating the Problem Matrix
Populating the matrix with every problem type that we face within the industry would create a very cluttered chart. One thing to recognize is that the wellbore is a VSC that we created to connect us to the reservoir. Anytime we lose control of how fluid enters the wellbore from the reservoir, we have a VSC.
Thus, we start by reviewing the more common problems associated with losing control of the wellbore such as casing leaks, packer leaks or plugs, mis-perforated intervals, cement channels, perforated wrong interval, etc. These problems all fall in the upper left quadrant. Since they are strictly void space or conduit flow, they are controlled totally at the wellbore.
The next example helps us understand the importance of geologic controls and understanding. If we have a watered-out layer with no crossflow between layers, this problem falls in the upper right quadrant, since the flow problem is dominated by permeable flow, but due to no crossflow between layers, all the control exists at the wellbore.
We then move to the more complex problem of a watered out layer, but one with extensive crossflow between layers. This problem would fall in the lower right quadrant as the flow problem is permeability related, but since there is extensive crossflow, there is really limited or no control at the wellbore.
These last two problems exist as a continuous grade from the upper right quadrant to the lower right quadrant, depending on the level of crossflow between layers.
The lower right quadrant contains all issues where reservoir permeability is very heterogeneous and/or anisotropic. That is where permeability can change directionally as well as in magnitude. Deltaic braided stream depositional environments can create this type of permeability variance. The key aspect of this problem is that this flow problem is totally dominated by permeable flow and all control is located well away from the wellbore.
A reservoir that is highly faulted and fractured and where fluid can easily move through those features would fall in the lower left quadrant. The problem flow exists within the void-space feature of the fractures, but since there is extensive natural fracturing, the control is dominated deep in the reservoir. These features can vary from extremely tight with limited transmissibility to very open and highly conductive. An induced fracture will be void-space flow, but to a limited extent, the control is somewhat more accessible from the wellbore. Karsted intervals (with connected vugular sections) are void-space flow problems, but their degree of control is based on how connected they are to vertical flow features (i.e., fractures or permeable rock) away from the wellbore. One other feature of these deep reservoir VSCs is that they often change in their conductivity over time due to dissolution or erosional enhancements.
In most problem scenarios, the ability to properly characterize flow is the biggest limitation to designing the best solution. Engineers usually equate any pressure drop over a region as a function of Darcy’s law and permeable flow. However, conduit flow (i.e., flow in large void-space features) might be better characterized by pipeline flow. The critical piece of this understanding relates to the nature and size of the void-space features. How we design solutions, or mixtures of materials to control these features, is closely related to the flow mechanism’s size, shape, and overall extent within the wellbore and the formation.
True permeable flow in competent rock, even up to several darcys, can be controlled by sufficient volumes of very strong gels. However, larger VSC features associated with enhanced faults or fractures, karsts, large wormholes, etc. will require a much stronger material, typically cements or very strong preformed particle gels designed as void-space fillers. We will discuss this more when we focus on the solution overlay, but for now, recognize that it is very important to properly characterize the nature of the flow to generate effective solutions.
Solution Types and Solution Continuum
Before we dive into the specifics about which solutions might work best for a specific set of characteristics, we must recognize that the solution options for conformance engineering problems range from a myriad of existing wellbore interventions to more extensive tailored solutions. This set of solutions can be represented by a solution continuum (Fig. 2).
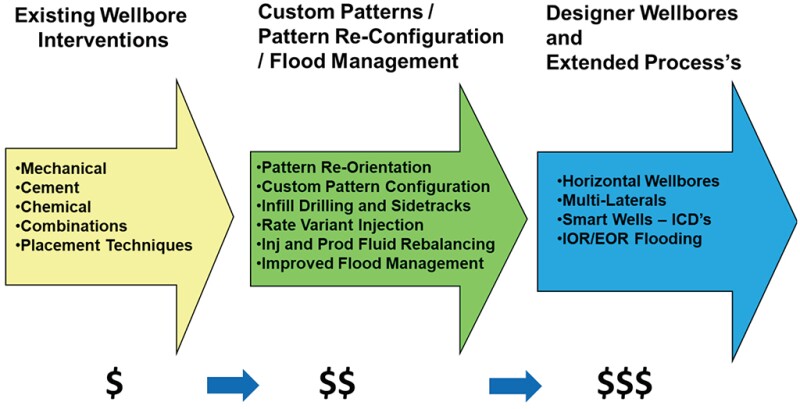
Distinguishing the best option within this continuum can be very difficult and often takes years of working on a given field or project to identify the most cost-effective approach. However, in most cases within the industry, we start with, and/or focus most of our time on, existing wellbore interventions. Let’s look more intently at existing wellbore Interventions.
Relating Solutions to the Problem Matrix
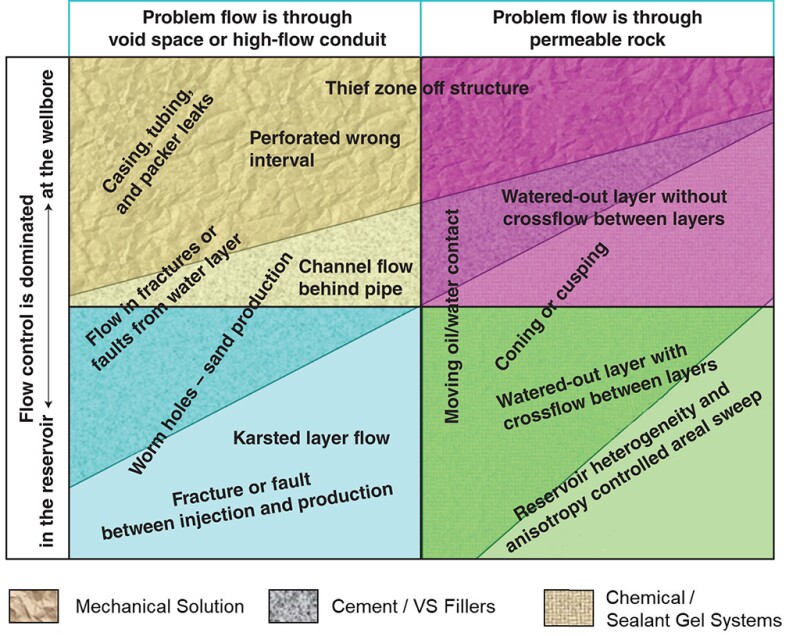
Now that we have a populated matrix with a variety of problems, how does this help us relate to an appropriate solution? With existing wellbore intervention solutions, I think we would all agree that keeping to the simplest solution is often best. In the case of several conformance issues, this typically means mechanical solutions, bridge plugs, straddle packers, scab liners, etc.
However, we know that these solutions are only effective in the upper half of this matrix. In certain situations, mechanical solutions may not be available due to the existing completion character. In those cases, we might be able to use cement or other void-space fillers (VSFs), and if the problem is in the upper right quadrant, chemical solutions such as permeability blockers can be used.
In the cases where the wellbore intersects another VSC and extends control away from the wellbore into the lower left quadrant of the problem matrix we can use cement and other VSFs. The key to creating effective solutions into the lower left quadrant is our ability to pump large volumes of VSF materials that have the proper strength to control that area. We can improve our effectiveness in these types of solutions by tapering the strength of the materials we use. A great example of this is found in SPE 103044. When we are limited to existing wellbore intervention solutions, we can only generate effective solutions for the lower right quadrant problems through the utilization of deep permeability-penetrating solutions or extremely small nanoparticle systems. These are included in the chemical solutions. We can further see this relationship between mechanical solutions, cements/VSFs, and chemical/sealant gel systems by using a graphical overlay image on the problem matrix (Fig. 3).
If this is all we had to do, the world of conformance engineering would be simple. However, in many cases, we end up with multiple problems in one well or field. For example, we have hydraulically fractured a well in a field with significant permeability streaks. Perhaps we have extended our fractured interval, thus connecting it to an aquifer. In some cases, we have karsted intervals combined with extensive fracturing, either induced or natural. In other situations, we have not controlled the wellbore connectivity to the void space or conduit flow, thus limiting our overall control (i.e., slotted liners, wire-wrapped screens, or pre-packed liners). In addition, we also have problems that may have started out as limited flow, but over the years these features get enhanced through additional rock dissolution or erosion. As discussed in Part 1, understanding these problems and their overall impact is critical to our ability to design effective solutions.
Designing an effective solution must focus on controlling, or at least aggressively influencing, the most dominant flow problem. In cases where a VSC is involved, this will always be the dominant feature. This is the one area where we have lucked out. Our ability to control void-space flow is considerably better than our ability to design and place solutions that gain significant control over permeable flow issues. Mechanical systems are the easiest to place and usually the easiest to remove if they don’t work. Cement and VSFs are next, and our experience with remedial (or squeeze) cementing often comes with many years of experience. The one significant problem with remedial cementing is that, for years, the industry has only focused on regaining control at or very near the wellbore, and today’s problems often demand that we influence these void-space conduits well beyond the wellbore.
There are also situations when multiple solution techniques can be implemented at one time to gain synergy from the two solutions. One example is pumping gels, followed by cement, as in gas-shutoff cases documented at Prudhoe Bay.
In addition to the papers on Prudhoe and Anton Irish, I recommend two papers on West Sak that utilize this technique and that show the progression of solutions that result from the performance evaluation and problem understanding improvements (SPE 169073 and SPE 201302).
Returning to the problem matrix with the solution overlay, none of these solution boundaries or problem placement locations are hard and fast. There are always gray areas within the problems and the solutions that can alter their location to some degree. In addition, remember that although we may have done something to control one problem, many times multiple problems exist that reduce the overall benefit of the selected solution. The effect of economics can shift these boundaries, as well as technological improvements and/or physical limitations of the wellbore configuration. In addition, although chemical and/or gel systems can reach farther into the lower right quadrant, there are often times when many problems in this area lend themselves to better solutions from pattern reconfiguration or designer wellbores, as discussed earlier. Fig. 4 provides a general perspective on the interaction of the conformance solution continuum with the problem matrix, along with a severity vs. likeliness plot.
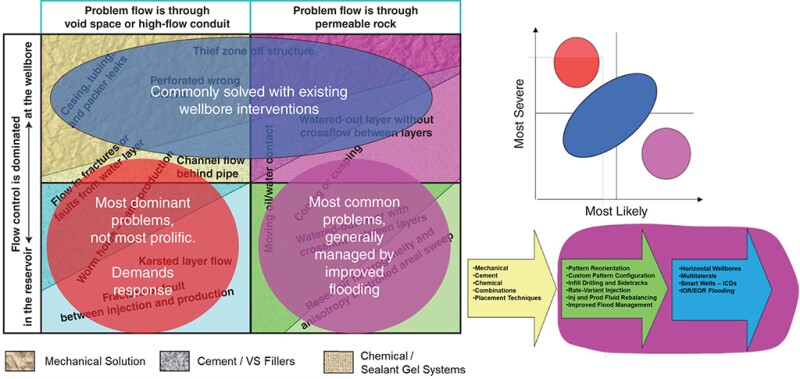
The perspective shown in Fig. 4 is provided not as a hard and fast relationship but more as a general rule that has developed out of multiple experiences, technical reviews, and learnings from others. It is my opinion that the greatest number of conformance problems that exist in the industry are related to permeability variance between zones that are significantly interconnected deep in the reservoir. These problems exist in the lower right quadrant and are very difficult to access and control from existing wellbores. These problems are not the most severe in terms of rapid or massive injectant cycling or breakthrough. The most severe problems result from direct VSC problems and require some form of physical intervention, or you lose the value of the wells involved.
Over the years, the permeability variance problems that exist in the lower right quadrant have generally been solved through sidetracking, custom pattern reconfigurations, injection/production rebalancing, etc. This is also the primary area where today’s application of big data analysis tries to correct these conformance problems through rebalancing of fluids.
To get the most value out of your conformance engineering efforts, you must continue to use the information you gain on each effort to learn even more about the problem and thus, formulate the best potential solutions for the conformance problems you encounter. Remember Step 5 of the process wheel in Part 1.
To have the greatest success rate in conformance problems you must revisit your problem understanding and the economics of the solutions applied.
Part 3 will focus on making sure that we are properly accounting for the benefits received from our diagnostic and solution efforts.
For Further Reading
SPE 84966 A Strategy for Attacking Excess Water Production by R.S. Seright, New Mexico Petroleum Recovery Research Center; R.H. Lane, Northstar Technologies Intl.; and R.D. Sydansk, Syndansk Consulting Services.
SPE 103044 The Successful Evolution of Anton Irish Conformance Efforts by D.D. Smith, M.J. Giraud, and C.C. Kemp, Occidental Petroleum, et al.
SPE 54596 Gel-Cement Combination Squeezes for Gas Shutoff by Q.J. Lai and A.J. Bond, ARCO Alaska Inc.; and T.W. Carpenter, ARCO AEPT, et al.
SPE 169073 An Overview of Conformance Control Efforts for the West Sak Field on the North Slope of Alaska by J.W. Peirce, M.R. Hutcherson, and M.D. Jensen, Conoco Phillips, et al.
SPE 201302 Case History of Conformance Solutions for West Sak Wormhole/Void Space Conduit With a New Reassembling Pre-Formed Particle Gel RPPG by G. Targac, C. Gallo, and D. Smith, ConocoPhillips, et al.

David Smith, SPE, is currently the president and principal advisor for Oilfield Conformance Consulting LLC and an adjunct professor for Missouri University of Science and Technology (MS&T). Prior to his current efforts and for approximately 20 years, Smith was the global conformance engineering advisor for either ConocoPhillips or Occidental Petroleum. Prior to that he was a project manager in conformance water management for Halliburton and held several positions within ARCO that were associated with profile modification and sweep improvement. Smith has been an active SPE member for more than 45 years. He was the technical program chairman for the 2014 SPE EOR/IOR Conference in Tulsa, a past co-chairman of the SPE EOR/IOR TIG (Technical Interest Group), and an SPE Distinguished Lecturer in 2019–2020. Smith holds a bachelor’s degree in geology from Pacific Lutheran University and an MS in petroleum engineering from Stanford University.

