Gas/Liquids Separators—Quantifying Separation Performance—Part 2

In this second article of a three-part series, methods for improved quantification of operating performances of the gas gravity separation, the mist extraction, and the liquid gravity separation sections of gas/liquid separators are discussed. These methods can be used for the selection and design of new separators, as well as the rating of existing separators.

Part 1 of the series in August provided a general discussion of separation equipment classification, as well as existing limitations to methods used for quantifying separator performance.

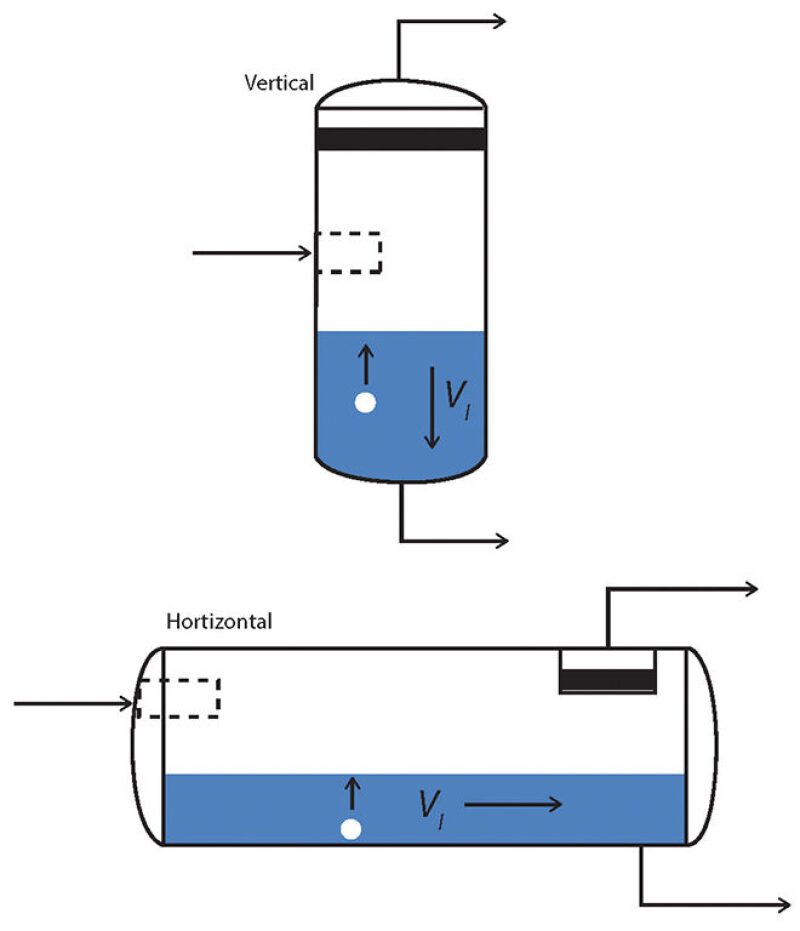
The main parts of a typical gas/liquid separator, vertical or horizontal, are shown in Fig. 1, including the feed pipe, inlet device, gas gravity separation section, mist extractor, and the liquid gravity separation section. Part 1 discussed the feed pipe and inlet device.

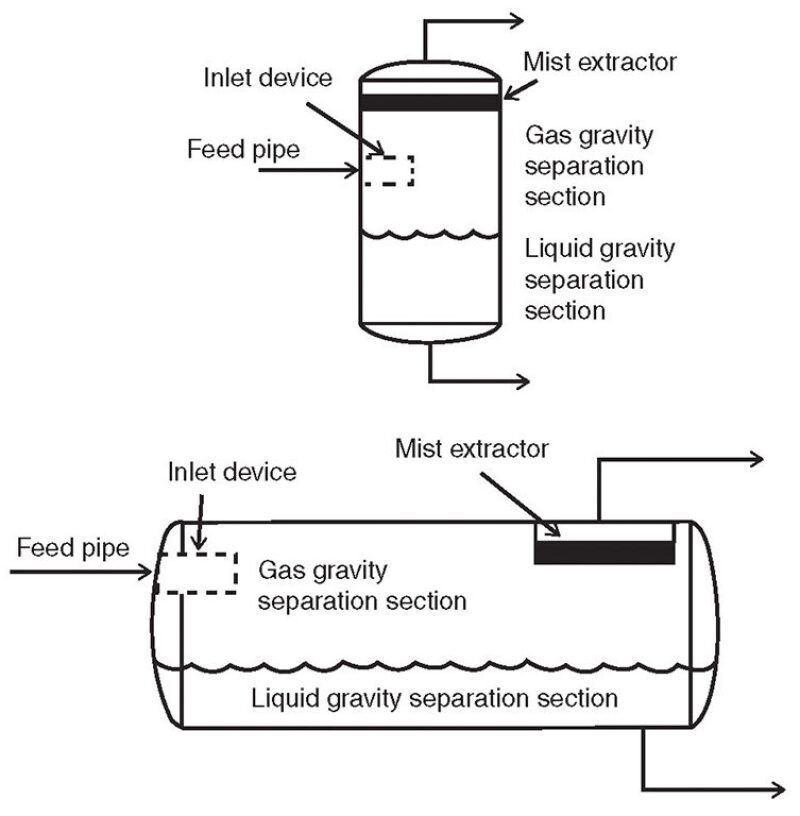
Gas Gravity Separation Section

The primary function of the gas gravity separation section of a separator is to reduce the entrained liquid load not removed by the inlet device. Available mist extractors have limitations on the amount of entrained liquid (droplets) that can be handled. A secondary, but related, function is the improvement/straightening of the gas velocity profile.

In low-liquid-loading applications, such as scrubbers, pre-separation of liquid droplets may not be required if the mist extractor can handle the entrainment load. However, even in this scenario, a relatively uniform gas velocity distribution should be delivered to the mist extractor to optimize its performance. The gas gravity separation section then provides preconditioning of the gas—and its entrained liquid load—ahead of the mist extractor.

Two approaches to sizing this part of the separator to remove liquid droplets from the gas are the K_s method (Souders-Brown equation as shown in Eq. 1) and the droplet settling theory.

The physical process is the separation of liquid droplets from the gas phase. Traditionally, the K_s method has been most commonly used because it usually provides reasonable results and is easy to use (K_svalues for vertical and horizontal separators are available from many literature sources). The method, primarily an empirical approach, involves the estimation of an allowable maximum gas velocity to achieve the required degree of droplet separation.

Souders-Brown equation:

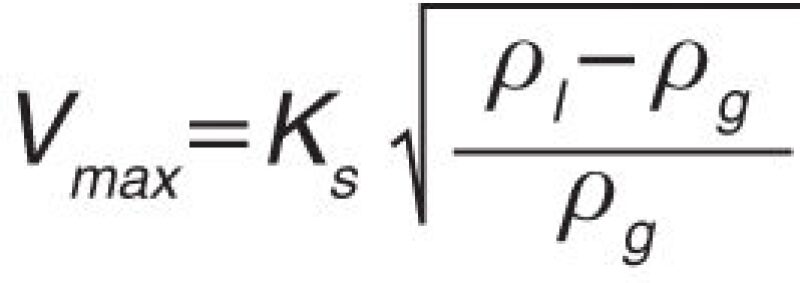
  (1)

 The K_s methodology does not readily lend itself to quantification of gas/liquid separation performance, so will not be discussed further.

After simplifying assumptions to make the calculations manageable, the droplet settling calculations for sizing the gas gravity separation section (cross-sectional area and length) aim at removing a target liquid-droplet size (e.g., 150 μm) and all droplets larger than the target size. Even this approach is only semiquantitative in that selection of an appropriate target droplet size is an inherently ill-defined procedure, and provides little indication as to the amount of entrainment remaining.

If we think of the gas gravity separation section as a preconditioning step ahead of the mist extractor, then it is clear that the requirements for separation performance of this part of the separator are dependent on the requirements of the selected mist extractor. Particularly important are the allowable liquid loading (gal/min/ft²) of the face area of the mist extractor and the droplet removal capability of the mist extractor.

The uniformity of the gas velocity profile through the mist extractor and limits on velocity to prevent re-entrainment are also important factors and will be discussed later.

The amount of entrainment and the gas velocity profile exiting the inlet device were determined by the method in Part 1 of the series in August 2013.

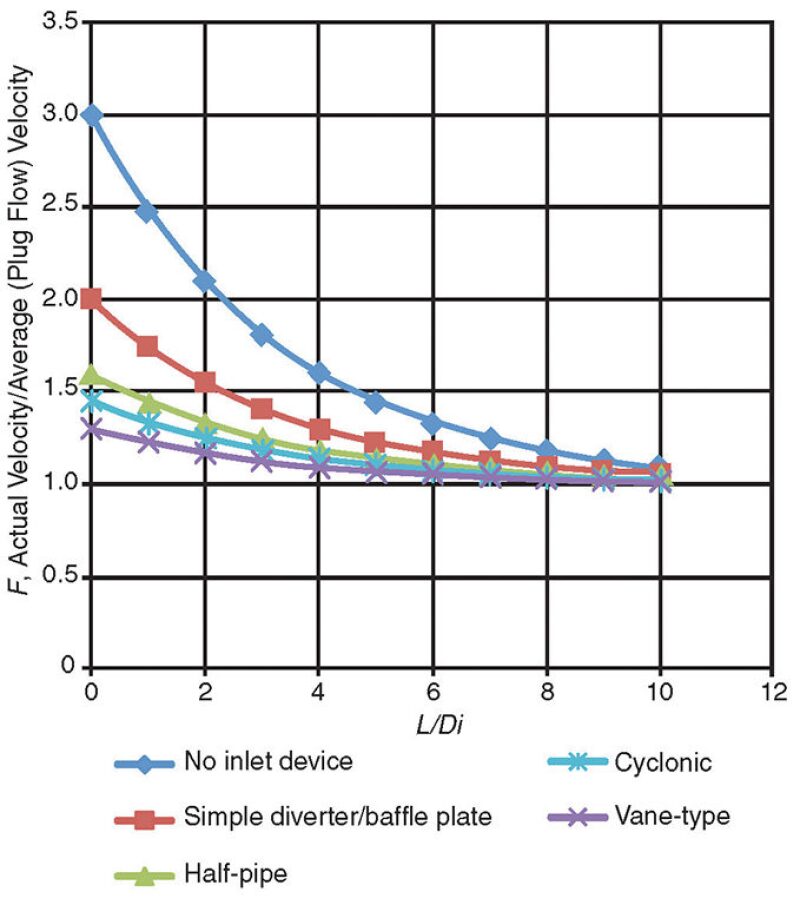
Fig. 2 shows the quality of the flow distribution immediately upon exit of the inlet device (L/Di=0) and the development of the flow profile with distance downstream of the inlet device. The quality of the flow distribution is characterized by the factor F, the actual average velocity/ideal plug flow velocity. F values greater than 1.0 imply unused cross-sectional flow area. Use of this factor will allow estimation of the effective actual velocity, which can then be used in the droplet settling calculations for the gas (and liquid) gravity sections. Note also that the calculated effective actual velocity for the gas gravity section will be the velocity at the entrance to the mist extractor section.

Release Point of Entrained Liquids at the Entrance to the Gas Gravity Separation Section

Fig. 3 shows the gas flow–droplet settling relationships for vertical and horizontal separators. With the droplet size distribution and effective actual gas velocity through the gas gravity separation section established, the droplet settling calculations are relatively straightforward for a vertical separator. For a horizontal separator, the effective release point of the droplets must be established before the settling calculations can be performed.

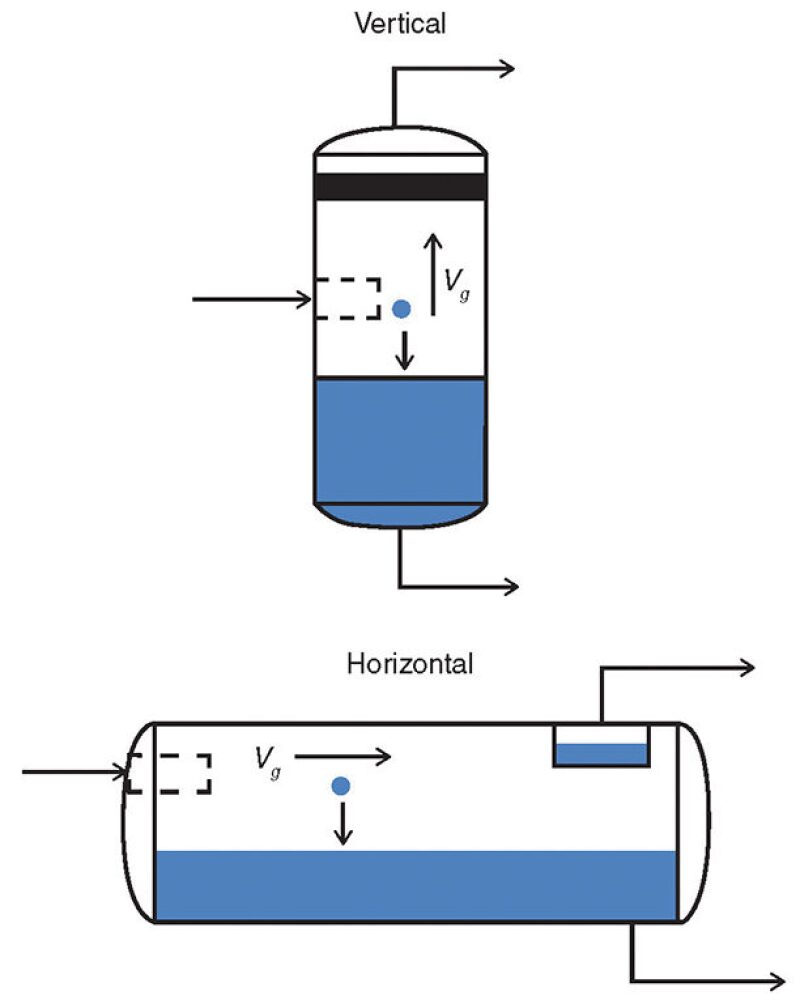
The geometry associated with the droplet settling calculations for a horizontal separator is shown in Fig. 4. The worst case (most conservative) assumption for the release point is that all droplets are released at the top (inlet) of the gas gravity separation section, as shown by the droplet settling trajectory in Fig. 4. A release point closer to the gas/liquid interface results in better calculated droplet-removal performance and a lower entrainment load exiting the gas gravity separation section (mist extractor inlet). The type of inlet device and its location has a bearing on the effective entrainment load release point at the inlet to the gas gravity separation section. But because of the relatively chaotic gas flow patterns expected to be exiting from the inlet device, regardless of the type, attempting to specify the vertical position/height of the release point as a point source is probably not warranted. Additional investigation of this issue via computational fluid dynamics (CFD) modeling is recommended.

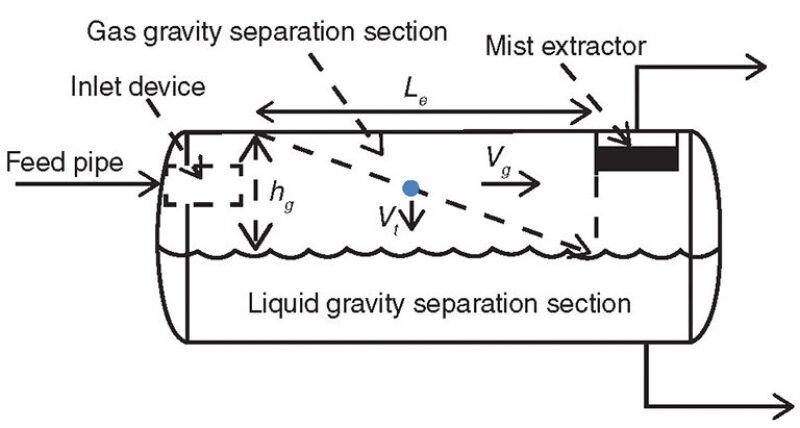
For the purposes of this article, it will be assumed that the release of entrained droplets occurs uniformly over the vertical height of the gas gravity separation section (h_g) in Fig. 4. This is a more optimistic assumption than the release point depicted at the top of the vessel in Fig. 4, but is more realistic. The actual release of droplets would be expected to occur over much of the cross-sectional area of the gas space at the inlet to the gas gravity separation section, which is less conservative than the assumption used here. The current release point assumption does not result in a sharp, separable droplet cutoff size, which is also more realistic.

Droplet Settling Calculations

Droplet settling calculations have typically been based on the separation of a target droplet size. The primary assumption is that if all droplets larger than the target droplet size are removed, the amount of entrainment remaining would be within the capability of the mist extractor.

The following discussion does not use this approach; instead, it is based on quantification of the entrainment loads, droplet sizes, and liquid-loading capabilities of mist extractors. Droplet/gravity settling theory is addressed in other references, so an overview of the subject will be provided here.

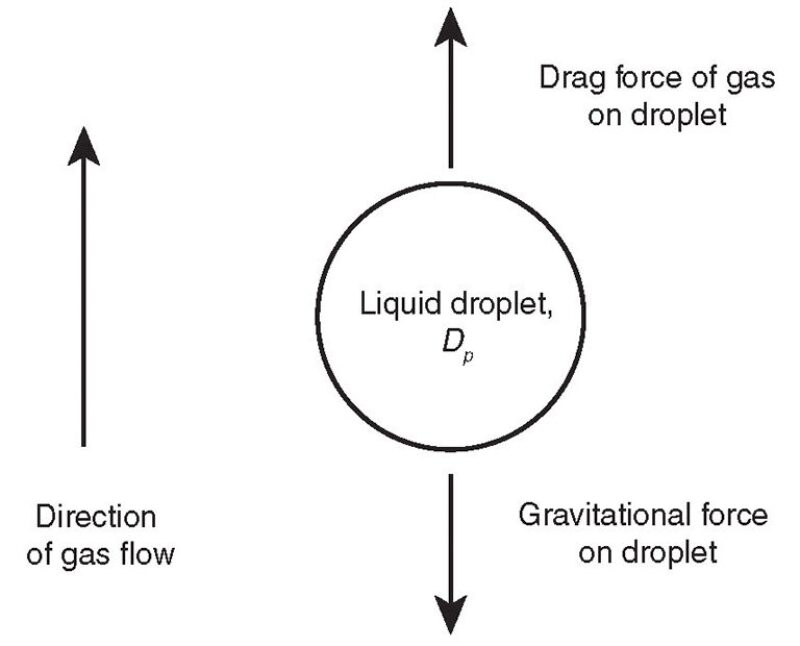
Eq. 2 represents a general form of droplet settling. It is obtained by performing a force balance on the droplet in a flowing gas stream, as shown in Fig. 5. These principles also apply to separation of gas bubbles from liquid and separation of droplets of one liquid phase from another liquid phase.

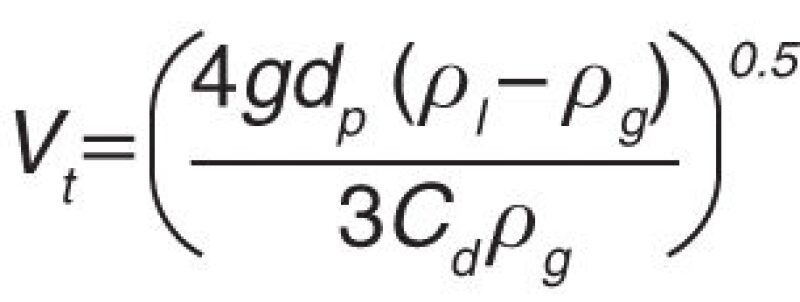
 (2)

The drag coefficient (C_d) is a function of the droplet/bubble Reynolds number, defined as:

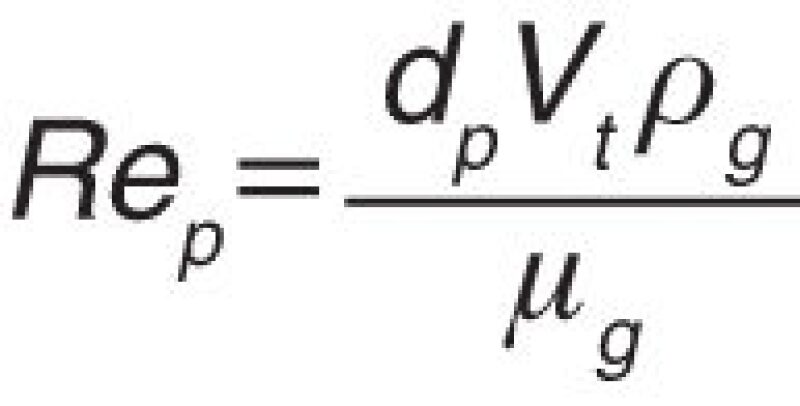
  (3)

The Reynolds number of the droplet includes the terminal velocity, which makes solving for C_d an iterative process, much like the solution for the friction factor in fluid-flow calculations.

Fig. 6 shows the relationship between C_d and Re_p for spherical droplets. The full range of Reynolds numbers for droplets can be divided into the regions associated with the laws of settling—Stokes’, intermediate, and Newton’s.

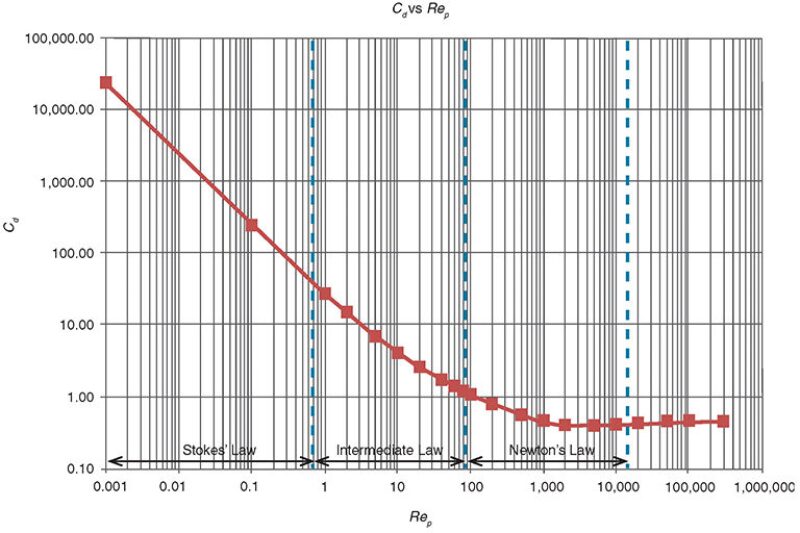
Table 1 shows the derived equations for terminal velocity for the three settling regions.

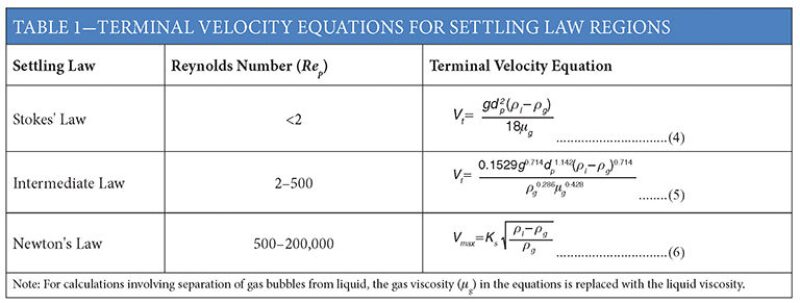
For the gas gravity separation section, the entrained droplet sizes of most interest will fall in the intermediate-law settling region. The very smallest and very largest droplets will fall into the Stokes’ and Newton’s law regions, respectively.

Vertical Separators

Using the droplet size distribution and effective actual velocity correlations discussed in Part 1 in August, the terminal velocity equation(s) mentioned earlier can be used to calculate the droplet removal efficiency of the gas gravity separation section. The results of this calculation will be the entrainment load (gal/MMscf) and the corresponding droplet size distribution at the inlet to the mist extractor. A sharp, separable droplet size cutoff will be obtained.

The F factor (Fig. 2) is needed to calculate the effective average gas velocity used in the terminal velocity equation(s) given in Table 1. Because of the relatively complex interrelationships between variables/parameters used in the quantitative approach discussed in this series of articles, an optimization algorithm is used to perform the iterative calculations.

Horizontal Separators

The calculations for the horizontal separators are more complicated than those for a vertical separator. Referring to Fig. 4, the residence time for the gas in the gas gravity separation section can be defined as:

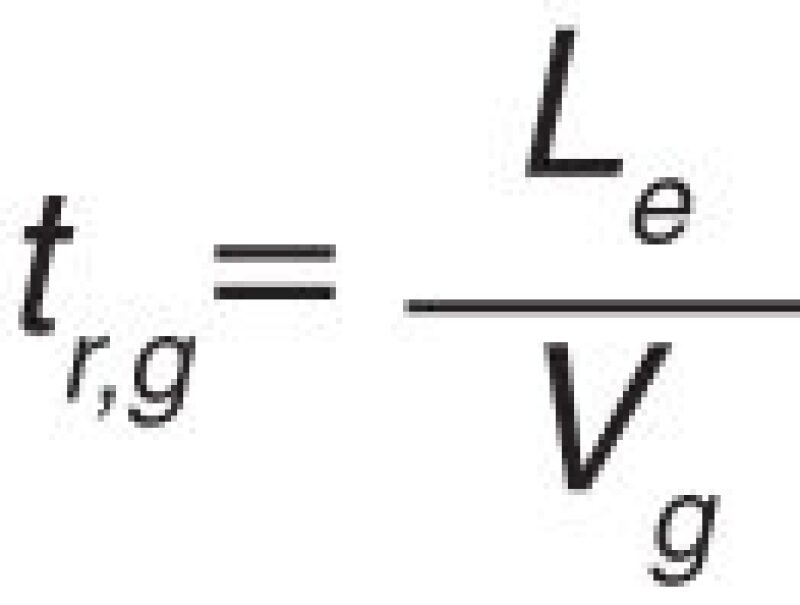
 (7)

A droplet of liquid is considered to be separated/removed from the gas if it falls vertically from its release point to the liquid level (h) within the time (t_r,g) it takes for the gas to traverse the horizontal length of the gas gravity section (L_e).

Equating the droplet drop time with the horizontal gas transit time gives:

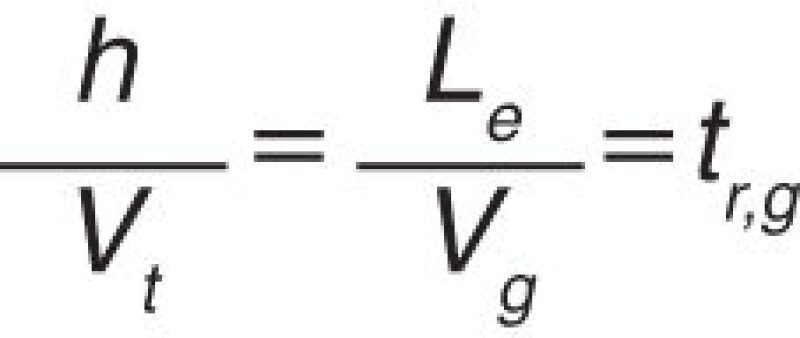
  (8)

If h is set equal to h_g, the vertical distance between the liquid level and the top of the vessel, the droplet trajectory shown in Fig. 4 is obtained. Eq. 8 can then be rearranged to give:

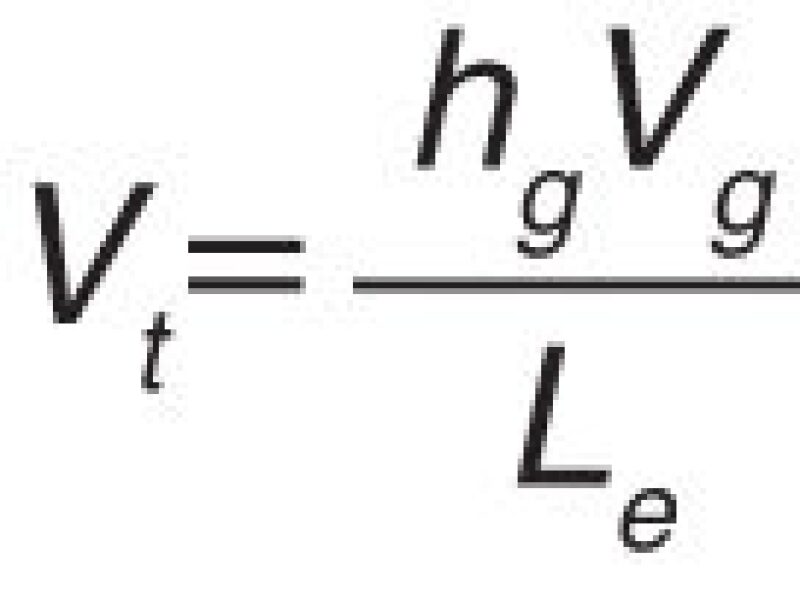
  (9)

This is the terminal (settling) velocity of the droplet corresponding to the trajectory shown in Fig. 4. The value for the terminal settling velocity can then be substituted into the appropriate settling velocity equation in Table 1 to determine the corresponding droplet diameter (d_p). This droplet size, along with all larger droplets, will be separated with 100% efficiency for the given conditions (d_p,100).

Because of the assumption made earlier that droplets are released uniformly over the vertical height (h_g) of the gas space, an additional calculation is required to determine the separation efficiency of droplets smaller than d_p,100.

Eq. 8 is rearranged to give:

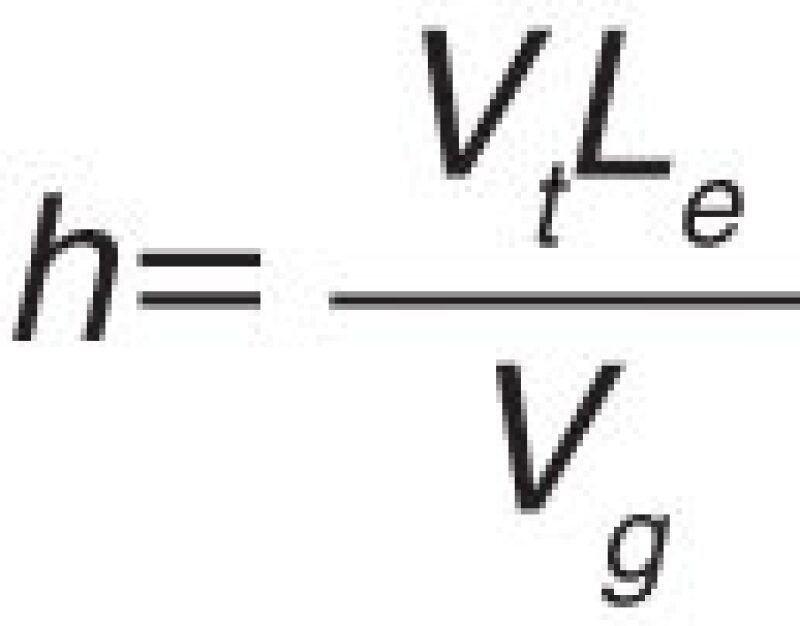
 (10)

For droplets smaller than d_p,100, terminal velocities can be calculated using the equations in Table 1. As droplet size decreases, the terminal settling velocities will also decrease, resulting in decreasing values for h, the maximum allowable release-point height that results in separation of the droplet. This leads to the following relationship between droplet size and removal efficiency for droplets smaller than the calculated d_p,100 size:

(11)

For the purposes of this article, the distance h_g and gas velocity V_g have been based on the liquid level at high-level shutdown, which may be conservative, but is intended to ensure liquid-removal separation performance over the entire range of potential operating conditions.

With these calculations applied to the entrainment load and its corresponding droplet size distribution, the separation performance of the gas gravity separation section can be quantified. The entrainment/droplets not separated are carried onward to the mist extraction section.

Re-Entrainment

For horizontal separators, there are limits to the velocity of the gas flowing across the surface of the liquid to prevent re-entrainment of the liquid into the gas phase. The equations for calculating re-entrainment conditions are similar to those developed by Ishii and Grolmes (1975) and have been adapted to apply to horizontal separators by Viles (1993).

The re-entrainment constraint, while not typically limiting, will be determined and used in separator sizing results to be presented in the next article in this series in December.

Velocity Profile Modifiers: Perforated Plates

Quantification of droplet separation in the gas gravity separation section of a vertical or horizontal separator requires an estimate of the effective actual gas velocity. The actual gas velocity will always be higher than the ideal plug flow velocity, making droplet separation more difficult. It is possible to modify the gas velocity profile and make it closer to the ideal plug flow. Typically, this is done by using flow straightening internals, often in the form of perforated plates. These plates use hole sizes of approximately 0.5 in. diameter with 20% to 25% open area.

For the purposes of this article, use of a perforated plate in the gas or liquid phase is assumed to provide a 50% improvement in the uniformity of the velocity profile, based on inspection of published CFD results. For example, if F = 1.6 without a perforated plate, use of the plate would result in an effective F factor of 1.6−0.5(0.6) = 1.3. This will reduce the effective average gas velocity and improve droplet separation efficiency.

Mist Extractor

The mist extractor acts as the final gas-cleaning step in a conventional separator. Its selection and design will determine the amount of liquid remaining in the separated gas phase (carry-over). Historically, and certainly for many onshore applications, “mist extractor” referred to a mesh pad. Mesh pads are commonly used, but higher-capacity mist extractors, which include vane-type (vane packs) and axial-flow demisting cyclones, are being increasingly used—especially in large-flow applications.

Fig. 7 shows the location and function of a typical mist extractor in a vertical separator.

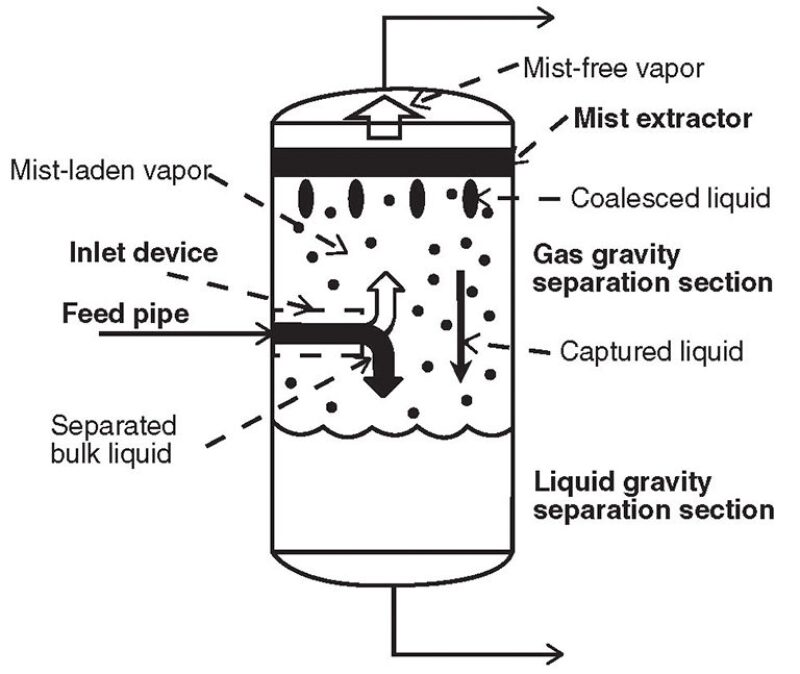
The purpose of the mist extractor is to remove the droplets of liquid remaining at the outlet of the gas gravity separation section.

Mist Extractor Performance

Mist extractor performance depends on the following:

• Droplet removal efficiency. The ability to remove smaller droplets will correspond to less droplet penetration through the mist extractor and, therefore, less carry-over of liquid into the separated gas. The required removal efficiency and tolerable amount of carry-over is determined by the sensitivity of the downstream process or equipment to the liquid content of the gas. Extremely sensitive processes, such as molecular sieve beds and amine contactors, likely will require a well-designed and properly sized coalescing filter, though some of the highest efficiency mist extractor designs can approach this level of droplet removal performance, and often with a more robust piece of equipment.
• Gas capacity. Gas capacity is usually determined by the maximum allowable velocity before re-entrainment becomes excessive. Capacity is typically quantified using the Souders-Brown equation (Eq. 1). Each mist extractor type has its own K_s factor, usually different from the values used to size the gas gravity separation section. Ideally, there should be correlations/equations that quantify K_s values for mist extractors as a function of the mist extractor design/construction details and the in-situ fluid properties and flow conditions. Although some information is available about this, further work is required. Most mist-extractor capacity information is based on low-pressure air/water tests, which do not scale up well to real-world conditions.
• Liquid handling. This refers to the amount of entrained liquid load (gal/min/ft²) that the mist extractor can handle before separation performance is substantially degraded (usually because of re-entrainment).

Secondary considerations include turndown performance and fouling tendency.

Mesh Pads

Droplet Removal Efficiency

The primary droplet capture mechanism used by wire-mesh mist extractors is inertial impaction. A methodology that can be used to quantify droplet capture efficiency of a mesh-type mist extractor is as follows:

1.  Calculate the Stokes’ number (sometimes called the inertial impaction parameter) from the following equation

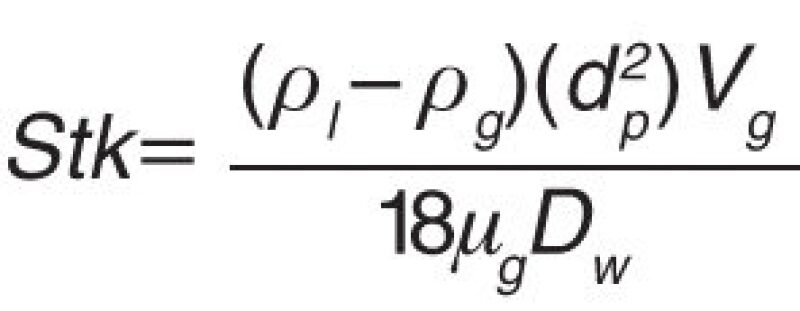
  (12)

(Note: Some literature sources define the Stokes’ number with a value of 9 in the denominator instead of 18.)

2.  Calculate the single-wire removal efficiency from Fig. 8 (Langmuir and Blodgett 1946).

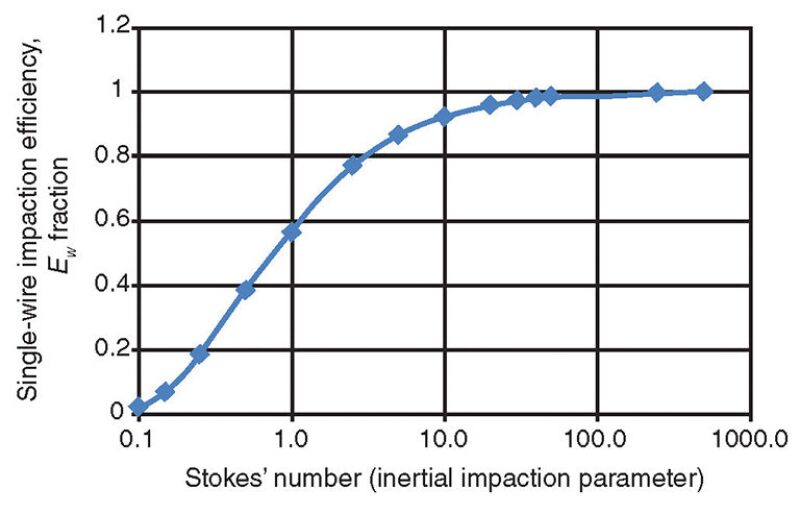
An approximate curve fit of Fig. 8 is given by the following equation:

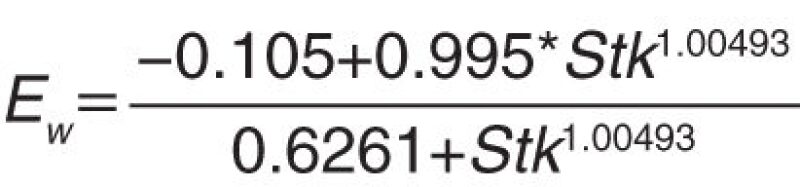
 (13)

3.Convert the single-wire capture efficiency into the mesh-pad removal efficiency using the following equation based on the work by Carpenter and Othmer (1955).

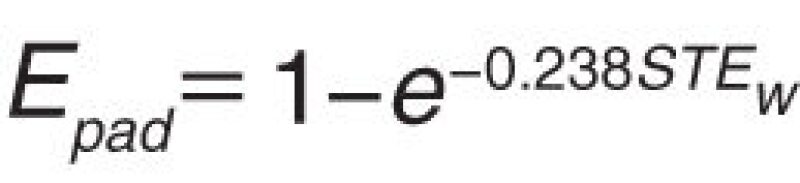
 (14)

Mesh Pad Capacity

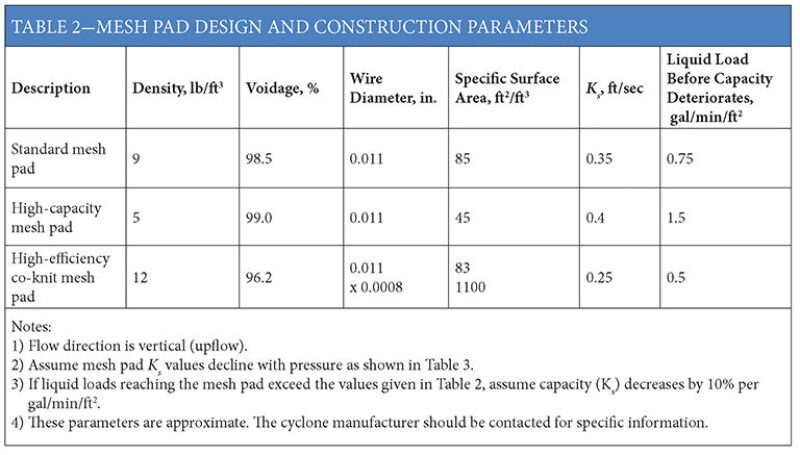
Mesh pad capacity is defined by the K_s value as used in the Souders-Brown equation. Information, including K_s values, for several mesh pad designs is provided in Table 2.

K

s

Deration for Pressure

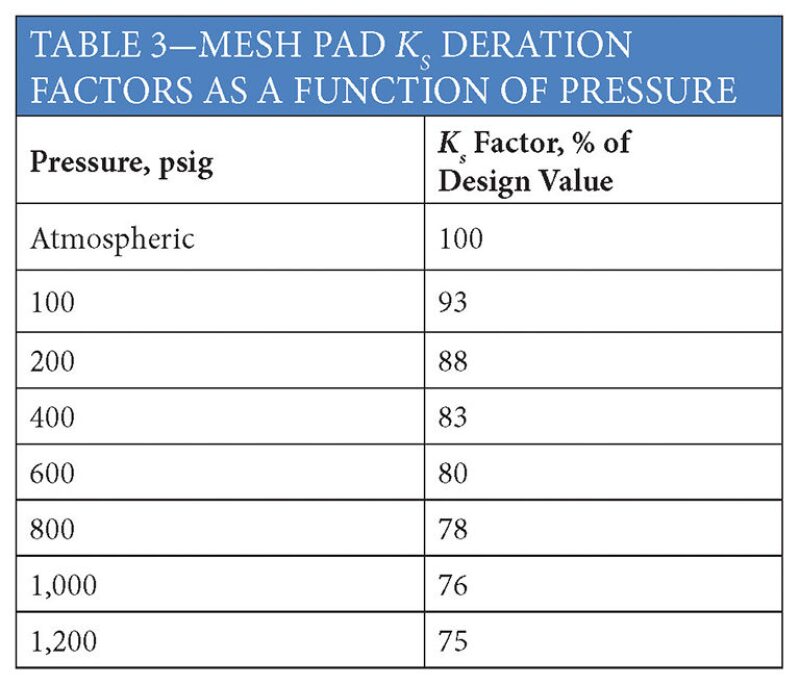
It has been found that the capacity of most mist extractor types, including mesh pads, declines with increasing pressure. This behavior is believed to be mainly due to the increasing gas density/liquid density ratio (ρ_g/ρ_l) and decreasing liquid surface tension that occurs with increasing pressure. Table 3 was originally developed for mesh pads, but is used as an approximation for other mist extractor types (Fabian et al. 1993).

Vane-Type Mist Extractors

Droplet Removal Efficiency

Similar to mesh pads, the primary droplet capture mechanism used by vane-type mist extractors is inertial impaction. The following equation (Monat et al. 1986) can be used to estimate droplet separation efficiency:

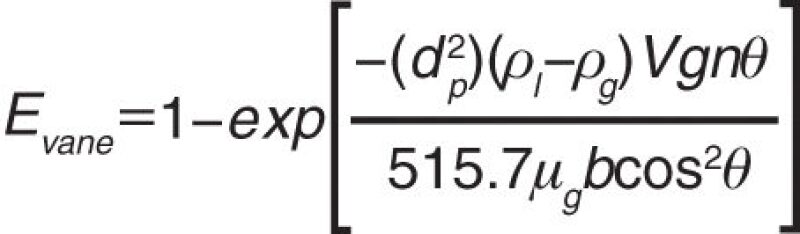
 (15)

This equation does not account for the effect of pockets, if present. It also includes a variation of the Stokes’ number defined earlier.

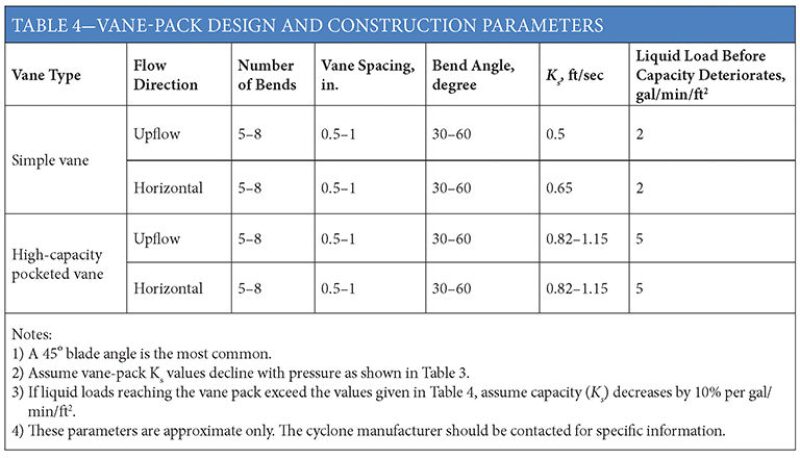
Table 4 provides construction and design parameters, including K_s values, for several generic vane-pack types.

Demisting Cyclones

Droplet Removal Efficiency

The following formula can be used for calculating the droplet removal efficiency of an individual axial-flow cyclone tube (Austrheim 2006).

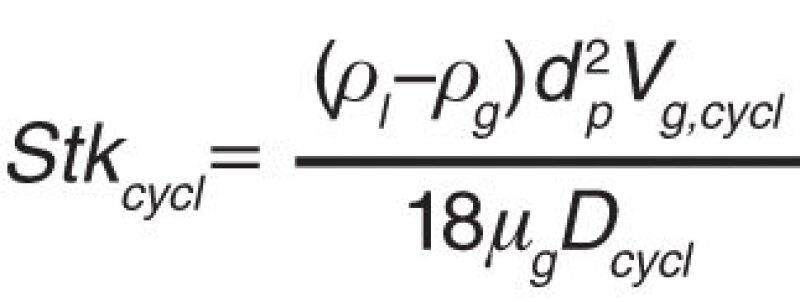
   (16)
V_g,cycl is the superficial gas velocity through a single cyclone tube. Given the in-situ volumetric gas-flow rate, to determine the velocity through an individual cyclone it is necessary to know the number of cyclones and their sizes (cross-sectional flow area/cyclone). This information should also correlate to the K_s value used to quantify capacity for a demisting cyclone bundle, based on bundle face area. For the purposes of this article, it is assumed that the individual cyclones are laid out on a square pitch pattern with a centerline to centerline spacing of 1.75D_cycl (cyclone inside diameter).

Fig. 9 provides an example of the droplet removal calculations using the equations given earlier.

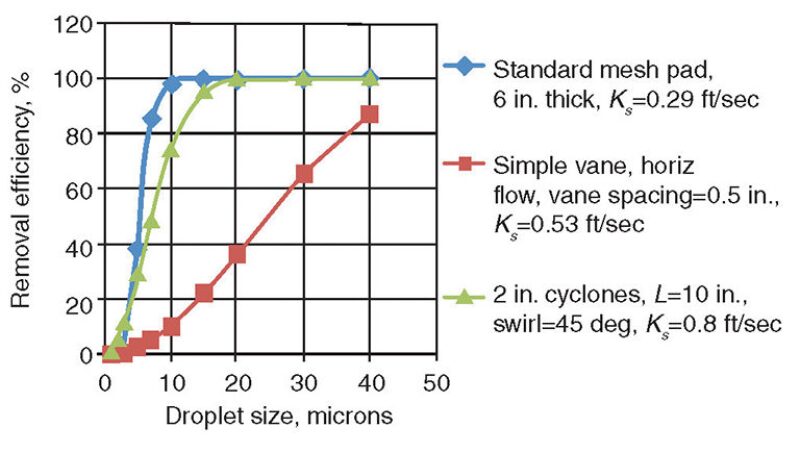
Use of Different Mist Extractor Types in Series

It is often advantageous to use two different types of mist extractors in series. The most commonly used arrangements are a mesh pad followed by a vane pack, and a mesh pad followed by a demisting cyclone bundle.

At low flow rates, the mesh pad does nearly all of the work to remove droplets. Mesh pads have good droplet removal efficiency and good turndown performance, generally speaking. Their main weakness is that they have elatively low capacity (K_s values) compared to other types of mist extractor. At high gas-flow rates, the mesh pad will be operating above its capacity limit and will be entraining (carrying over) liquid from its downstream face. However, it has been found experimentally that the re-entrained liquid exiting the mesh pad is in the form of droplets substantially larger than those at the inlet to the mesh pad. When operated above its capacity, the mesh pad acts as a coalescer, shifting the entrainment droplet size distribution to larger sizes. These larger droplets are easier to remove by the secondary downstream vane-pack or demisting cyclone bundle. The mesh pad provides good low-flow droplet removal performance, while the secondary vane-pack or demisting cyclone bundle provides high-flow capacity with improved droplet removal performance as a result of the larger droplets exiting the mesh pad.

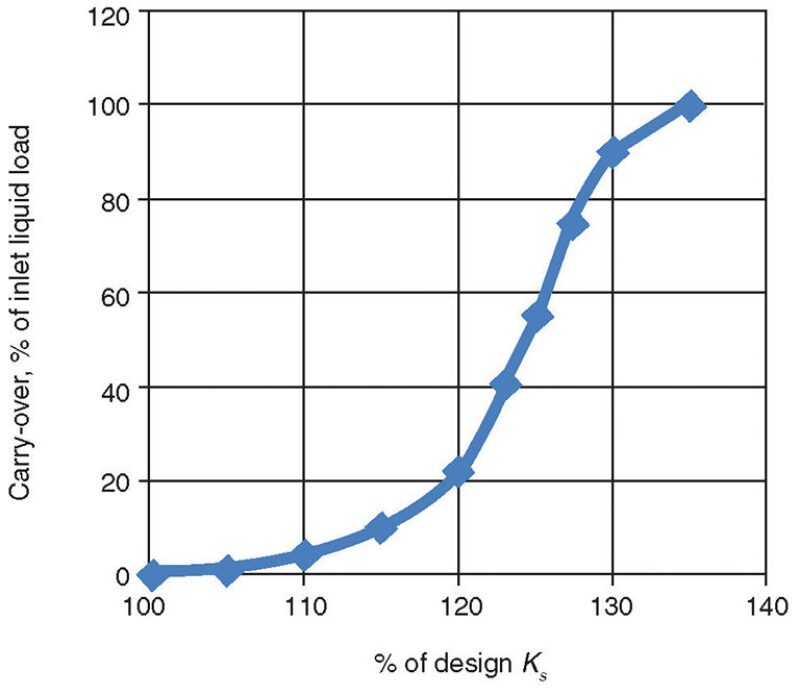
It is possible to quantify the performance of the series mesh pad configuration by estimating how carry-over varies with K_s values above design for the primary mist extractor (usually a mesh pad) and how the droplet size is shifted by the mesh pad when operating above its re-entrainment point.

Fig. 10 shows the assumed approximate relationship between the percentages of carry-over vs. the percentages of design K_s. For simplicity, this article assumes an adjustable shift factor of 2 that is applied to the droplet size distribution calculated at the inlet to the primary mist extractor.

Liquid Gravity Separation Section

The functions of the liquid gravity separation section depend on the type of separator and its application, including the following:

1. Degassing of the liquid.
2. Smoothing out of intermittent inlet flow surges to provide steadier liquid flow to downstream equipment/processing.
3. To maintain a liquid seal at the bottom of the separator, a minimum requirement for instrumentation layout and process control.

Degassing of the Liquid

This process separates the free gas out of the liquid (oil) to prevent excessive gas carry-under. Associated issues include the following:

• Degassing does not usually control the gas carry-under, especially in high-pressure gas/oil separators.
• Degassing can be an issue for cold, high-viscosity heavy oil.
• Historically, liquid residence time guidelines (for example, API 12J 1989), have been used to size the liquid-holding part of the separator for degassing purposes. A more accurate and consistent method is to use the droplet settling theory equations presented earlier to design the dimensions of the vessel to achieve removal of entrained gas bubbles. As with liquid droplets in the gas phase, the difficulty is estimating how much gas is entrained in the liquid and the size distribution of the bubbles.

Many, if not most, separator applications will be dumping their recovered liquid to a lower pressure separator/process system via a level-control valve. By definition, the liquid in the separator upstream of the level-control valve is at its bubblepoint and, therefore, a significant amount of dissolved gas will flash out of the liquid as the liquid takes the pressure drop acrossthe valve.

The volume of this flash gas will typically make the volume of entrained gas bubbles look small in comparison. For this reason, sizing of the liquid handling section of a two-phase separator to remove tiny gas bubbles is usually not warranted. It should be noted, however, that there are separator applications where degassing is the controlling criterion.

If degassing of the liquid phase is the controlling criterion with respect to liquid handling, residence time and droplet (bubble) settling calculations are commonly used approaches. The approach using droplet settling calculations is discussed because it lends itself better to a quantitative analysis methodology.

Droplet (bubble) settling calculations

Droplet (bubble) settling calculations for the gas gravity separation section have historically been based on sizing for the removal of a target bubble size, such as 200 to 300 µm, with the assumption being that the resulting liquid would contain less than, say 1%, free gas by volume.

There has been little work done to estimate the amount of entrained gas in the liquid at feedpipe conditions, and even less research on the effect of the inlet device on gas entrainment in the liquid. There has, however, been research performed on the amount of gas entrained into a pool of liquid caused by a plunging liquid jet (the waterfall effect, as shown in Fig. 7), which can be significant.

Less work has been performed on quantification of the bubble size distribution associated with the entrained gas, but there is some guidance in this area. (The equations associated with these calculations are not included in this article. Bin (1993) provides the details.)

Quantification of gas entrainment into liquid by a plunging jet

With some simplifications, the plunging-jet equations can be adapted to separator applications by doing the following:

1. Estimate the effective liquid-jet nozzle diameter.
2. Establish the length of the jet, which is typically the distance from the inlet device outlet to the separator liquid level.
3. Calculate the effective jet velocity at the point where the jet enters the liquid pool.
4. Calculate the jet Froude number.
5. Calculate the amount of gas entrained into the liquid pool by the jet.
6. Calculate the depth of penetration of bubbles by the plunging jet (the release point of the bubbles).
7. Estimate the bubble size distribution.
8. Perform the bubble size separation calculations based on the separator geometry, including whether vertical or horizontal, the flow rates, and fluid properties. The calculations are analogous to those used for liquid droplet settling in the gas gravity separation section (Fig. 11).

The type of separator inlet device has an effect on the characteristics of the plunging liquid jet and, thus, the amount of gas entrained into the liquid in the bottom of the vessel. Inlet devices like the simple diverter plate and half-open pipe that do not distribute the bulk liquid phase over a large area, and which also direct the liquid downward, should be expected to entrain more gas into the liquid phase.

In a vertical separator, adjusting the liquid residence time by varying the vertical shell height containing the liquid will have a minimal effect on gas bubble separation performance, because height has a limited effect on liquid velocity in a vertical separator. On the other hand, varying the diameter will affect the vertical liquid velocity and, therefore, also will impact gas bubble separation performance. This is an example of a limitation of the residence time methodology and why it is necessary to understand the physical principles involved.

In the gas gravity separation section, perforated plates can be used to straighten out the velocity profile of the liquid. This is beneficial for those separator applications where degassing of the liquid is important. Otherwise, perforated plates in the liquid phase are normally used for liquid-liquid separation applications.

Handling Intermittent Flow

For many separator applications—in particular, the first separator at the outlet of a pipeline/gathering system, or a wellsite separator—the handling of intermittent flow should be the controlling criterion for sizing the liquid handling section of the separator. Unfortunately, liquid residence times of as low as 1 to 2 minutes are often used instead, which provides little chance for a separator to accommodate intermittent/slugging feed flow. The result is a separator that is constantly hitting high- and low-level alarm points, with occasional high- and low-level shutdowns.

The difficulty lies in the characterization of the inlet flow (the determination of gas and liquid flow rates vs. time on an hour-to-hour, if not minute-to-minute, timescale). The inlet flow characteristics are a function of the design and operation of the upstream feed system (Fig. 12).

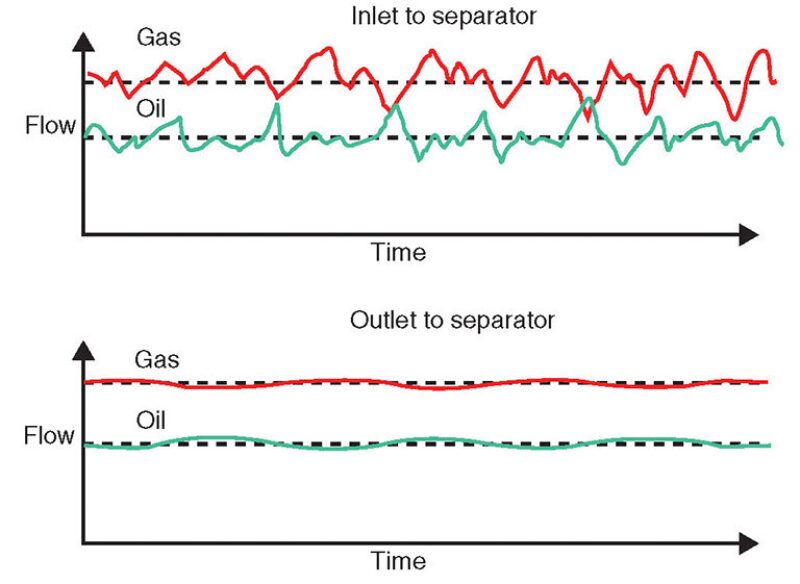
Liquid flow surges are accommodated by allowing the liquid level in the separator to rise and fall while holding the liquid outflow relatively constant. This requires level controllers that are tuned to allow the level to move up and down within the alarm setpoints. To smooth out gas flow rate surges, it is necessary to let the separator pressure rise and fall while holding the gas outflow relatively constant. If the swings in gas/liquid flow are large, as in cases where the fluids are received from a long, large-diameter multiphase pipeline, a dedicated slug catcher should be installed to attenuate the flow variations.

If the slug/surge volumes cannot be quantified in advance with a multiphase flow simulator, for example, the following approaches provide allowance for intermittent feed flow behavior:

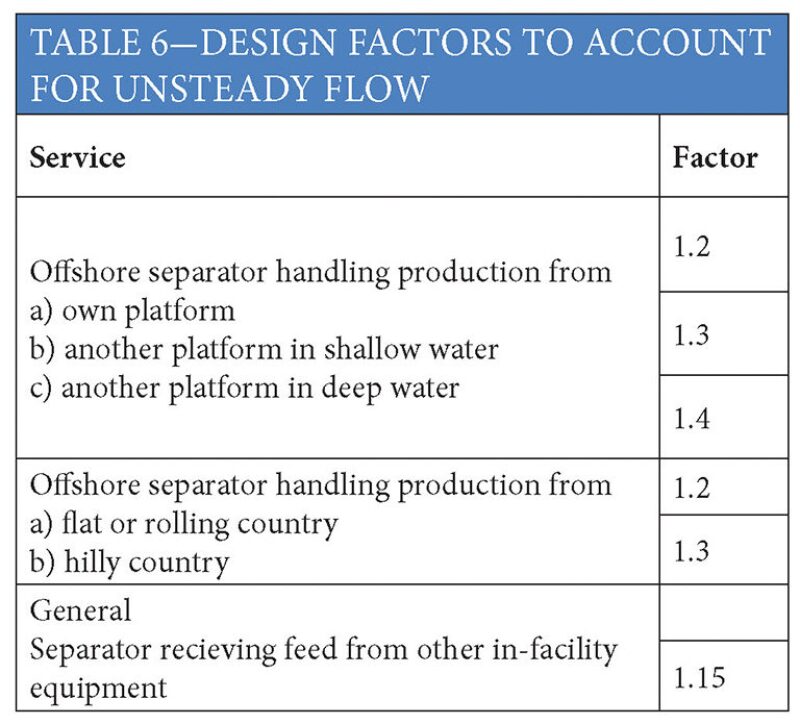
1. Inflate the steady-state flow rates, and size the vessel using standard procedures. Table 6 provides typical flow-rate multipliers based on the upstream feed supply configuration.

   

    
   

2. Estimate the slug/surge volumes. This requires an understanding of the various mechanisms that cause slugging and quantification of the slug/surge volumes involved. In the absence of better information, the slug size can be assumed to be from 3 to 5 seconds of liquid-full flow at feedpipe velocity. In-plant separators downstream of the inlet separation equipment would not be expected to see large slugs. A slug size based on 1 sec of liquid-full pipe at feed-flow velocity seems reasonable for these separators.

It is not possible to make generalized recommendations for separator performance quantification in relation to the handling of intermittent feed flow because there are too many variables involved. However, for specific applications, where many of the variables can be determined to some degree, more effort needs to be made than has typically occurred in the past.

Maintaining a Liquid Seal and Layout of Instrumentation and Control

The following criteria are typically used for layout/spacing of level-control instrumentation (Fig. 13):

• Minimum distances between alarm and shutdown settings for instrument connections/spacing.
• Minimum distances (volume) for stable level-controller action.
• Minimum distances (volume) to allow time for operator intervention.

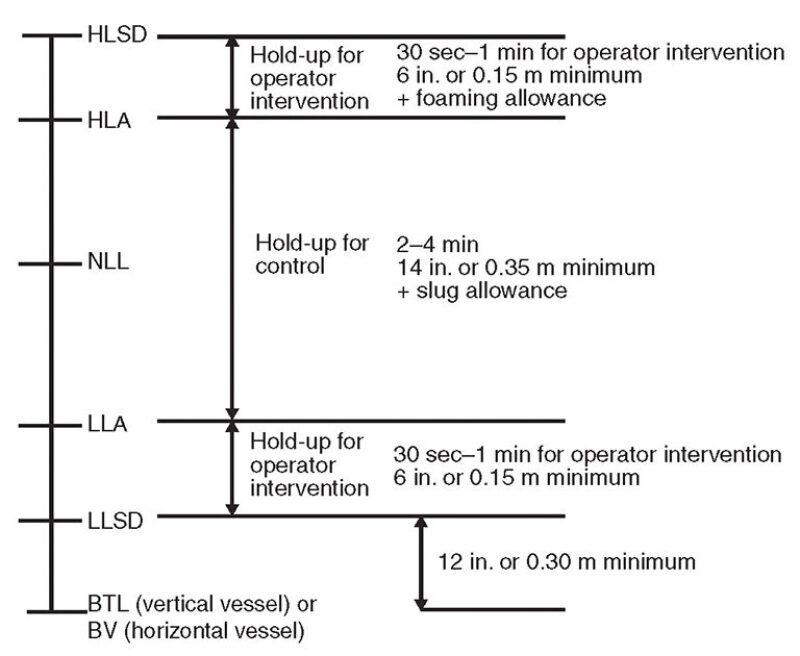
The times between alarm and shutdown shown in Fig. 13 are on the aggressive side compared to historical, and mostly onshore, guidelines. Intervention time requirements depend on a variety of factors, including the cause of the deviation, the facility’s control system, the operator staffing arrangement, and facility layout. If the separator has been designed properly, alarms should be infrequent. Longer times between alarm and shutdown mean bigger, more expensive vessels, and larger hydrocarbon inventories. 

Nomenclature
b = vane spacing, ft
C_d = drag coefficient, –
D_cycl = cyclone inside diameter, ft
D_p = droplet diameter, ft
D_p,100 = droplet removed with 100% efficiency, ft
D_w = wire or fiber diameter, ft
E_pad = mesh pad removal efficiency, Fraction
E_w = single-wire capture efficiency, Fraction
g = acceleration due to gravity, ft/sec²
h = release point height above liquid surface, ft
h_g =   gas space height for a horizontal separator, ft
K_s = Souders-Brown coefficient, ft/sec
L_cycl = cyclone length, ft
L_e = effective separation, ft
n = number of bends, –
S = pad-specific surface area, ft²/ft³
Stk = Stokes’ number, –
Stk_cycl = cyclone Stokes’ number, –
T =   pad thickness, ft
t_r,g = residence time of the gas, sec
V_g = gas velocity, ft/sec
V_g,cycl =   superficial gas velocity through cyclone tube, ft/sec
V_max = maximum allowable gas velocity, ft/sec
V_t = terminal settling velocity, ft/sec       

Greek Letters
a = cyclone inlet swirl angle, degrees
μ_g =   gas viscosity, lb/ft-sec
r_l = liquid density, lb/ft³
r_g = gas density, lb/ft³
μ_g =   gas viscosity, lb/ft-sec
q = bend angle, degrees

For Further Reading

API 12J, Specification for Oil and Gas Separators. 7th edition. 1989. Washington, DC: American Petroleum Institute.
Austrheim, T. 2006. Experimental Characterization of High-Pressure Natural Gas Scrubbers. PhD thesis, University of Bergen, Norway (September 2006).
Bin, A.K. 1993. Gas Entrainment by Plunging Liquid Jets. Chem. Eng. Sci. 48 (21): 3585–3630.
Carpenter, C.L. and Othmer, D.F. 1955. Entrainment Removal by a Wire-Mesh Separator. AIChE J. 1 (4): 549–557.
Fabian, P., Cusack, R., Hennessey, P., Neuman, M. 1993. Demystifying the Selection of Mist Eliminators, Part 1: The Basics. Chem. Eng. 11 (11): 148–156.
Ishii, M. and Grolmes, M.A. 1975. Inception Criteria for Droplet Entrainment in Two-Phase Concurrent Film Flow. AIChE J. 21 (2): 308–318.
Langmuir, I. and Blodgett, K.B. 1946. A Mathematical Investigation of Water Droplet Trajectories. Technical Report No. 5418, US Army Air Forces, Washington, DC.
Monat, J.P., McNulty, K.J., Michelson, I.S., Hansen, O.V. 1986. Accurate Evaluation of Chevron Mist Eliminators. Chem. Eng. Prog. December: 32–39.
Viles, J.C. 1993. Predicting Liquid Re-Entrainmen in Horizontal Separators. J Pet Tech 45 (5): 405–409.